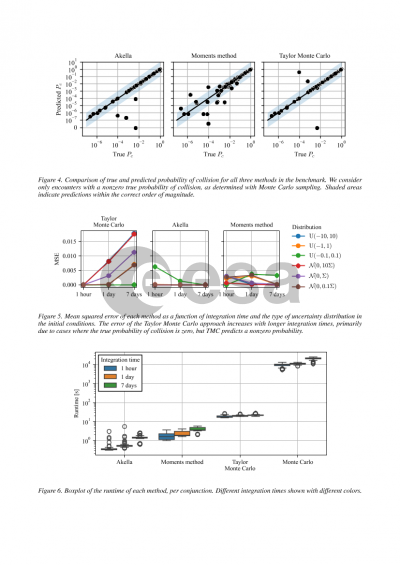
Document details

Abstract
Estimating the probability of collision between spacecraft and space debris is the first mandatory step for managing risks in space operations and designing effective collision-avoidance strategies. With the growing density of debris in key orbital regions, a precise yet efficient approach to compute collision probabilities is needed to safeguard active satellites and space infrastructure. Collision probability computation methods are generally divided into two categories: those tailored for short-duration encounters and those for long-duration encounters. While analytical approaches exist for both, they frequently assume Gaussian uncertainties and rely on simplifying approximations, which may introduce significant errors in highly nonlinear scenarios. To address these limitations, we build on recent advancements that enable the propagation of high-order statistical moments of the initial uncertainty without imposing a Gaussian assumption. We use high-order multivariate Taylor polynomials to represent the flow of the dynamics and compute what we call "event transition tensors" at the point of closest approach. This approach maps the statistical moments from the initial conditions to the event at a variable future time, creating a flexible framework that extends beyond fixed-time predictions. However, converting these propagated moments into a probability density function (PDF), and ultimately into a probability of collision, poses an additional challenge. To address this, we go beyond the moment-propagation methodology and derive an analytical expression for the PDF of the closest approach distance during an uncertain conjunction. Our method infers the PDF from the statistical moments using orthogonal polynomials, yielding an analytical yet computationally efficient solution for computing probabilities in uncertain scenarios. This process does not rely on strong simplifying assumptions (e.g., Gaussian distribution, long or short-term encounters, etc.), thereby making the method general in nature and applicable to any dynamical system, also beyond that of satellite collisions. We test the technique on 12 short-term encounter scenarios in low-Earth orbit discussed in the literature, with varying uncertainties, as well as on long-term encounters for geostationary satellites. Through these test cases, we show that our method accurately estimates the probability of collision in a number of scenarios where nonlinear dynamics and irregular distributions are prevalent, such as in highly elliptical orbits, encounters with small relative velocities, or non-Gaussian uncertainties. This is an important contribution in the field of space situational awareness, as it makes no assumption on the distribution of uncertainty or the encounter duration, unlike most current approaches. As a result, our method offers a versatile and accurate solution for collision probability estimation in complex dynamical environments.
Preview