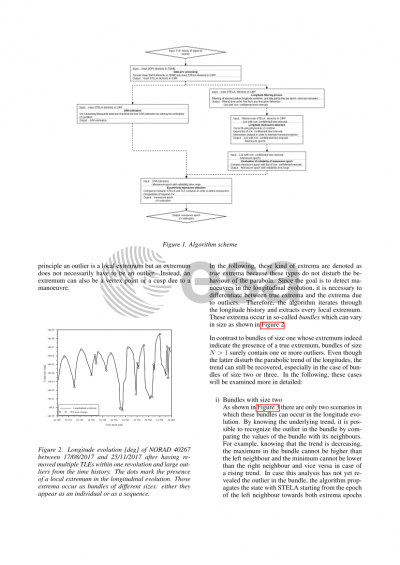
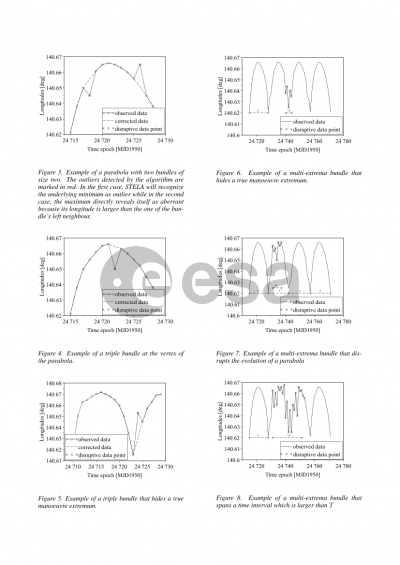
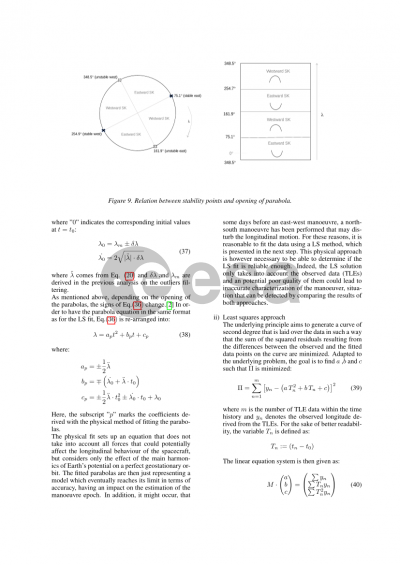
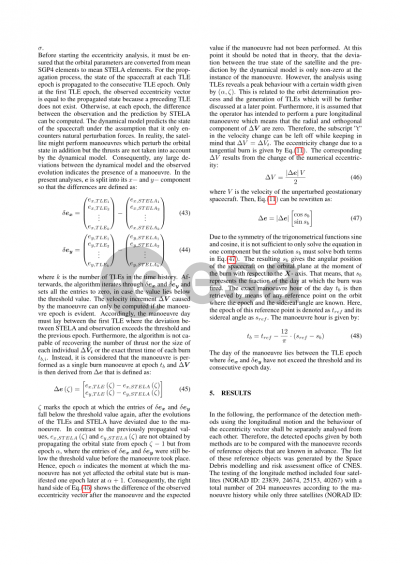
Document details

Abstract
The number of man-made space objects is dramatically growing nowadays. The continuous monitoring and studying of these objects are necessary to keep their number under control and ensure safe space operations. With respect thereto, international guidelines recommend decongesting the most populated space regions from satellites arriving at the end of their operational lifetime by performing post-mission disposal (PMD) strategies. Being able to assess the efficiency of PMD strategies is then essential to determine the guidelines compliance. For that it is required to detect when a satellite becomes inactive. In general, a satellite is considered to be functional if it is still performing periodic maneuvers to stay within the orbital operation configuration. This paper presents a promising method to detect historical in-plane maneuvers of satellites on a geostationary orbit (GEO). Since a maneuver changes the orbital state of the spacecraft, its effect can be detected by comparing the observed data to a reference evolution [1]. The reference model is represented in this case by the dynamical model STELA based on a semi-analytical theory [2]. The observed data is provided by the public American space object catalogue [3]. The TLE database contains the orbital state of each tracked object, however, not all six orbital parameters are interesting to study in terms of in-plane maneuvers. The evolution of the longitude and of the eccentricity vector is immediately affected by a maneuver that changes the shape or the size of an orbit. Within the longitude analysis, the maneuver epoch is estimated by focusing on the maneuver strategy. An operational spacecraft usually performs a maneuver as soon as the longitude motion threatens to violate the operational deadband. Consequently, the longitude evolution follows a parabolic motion [4]. Two polynomial curves of second degree are laid over the observation: the first curve is derived from a simplified dynamical model and the second curve is directly obtained through a Least Squares (LS) fitting method. The discrepancy between the LS and physical fitted parabolas gives an indication on the quality of the input data, the TLEs. The detected maneuver epoch must be companioned by a confidential parameter that denotes the time range around the estimated epoch in which the maneuver is expected to have happened. The maneuver interval is then forwarded to the eccentricity analysis where the maneuver epoch is estimated more precisely by studying the divergence between the observed and expected eccentricity vector evolution. The latter is propagated with STELA after having estimated the area-to-mass ratio that is needed in order to model the perturbation forces accurately. As soon as the observed eccentricity vector deviates significantly from the expected evolution, the epoch and the velocity V of the maneuver can be recovered, too.
Preview