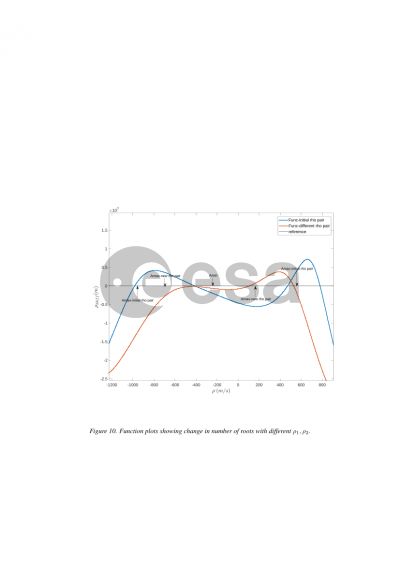
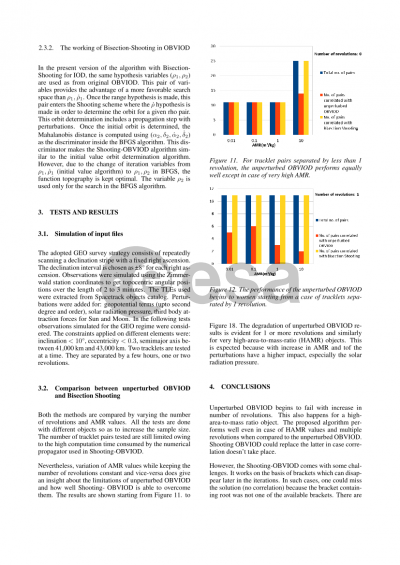
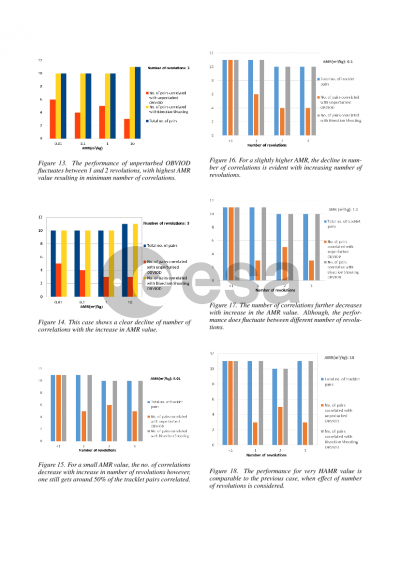
Document details

Abstract
Space debris is becoming a major threat for the functional spacecraft. Out of the thousands of tracked objects in the Earth’s orbits, only a small percentage corresponds to the operational satellites. It is clear that this problem needs to be addressed immediately. The first step towards solving it requires the monitoring of the space environment and cataloguing the debris. Optical surveys result in angles-only observations of objects on very short arcs. These observations are sparse and cover a very small part of the orbit, hence the initial orbit determination becomes challenging. Two observation series are associated together to find out if they belong to the same object and an initial orbit is computed. One way to do it is by using the Optimized Boundary Value Initial Orbit Determination (OBVIOD). This algorithm does not include perturbations. To include the latter we use a so-called shooting method. The shooting scheme is a boundary-value approach, which works like an initial-value method. It takes a hypothesis at the initial boundary and propagates it to the second boundary, where the computed value and the original boundary value are compared. The hypothesis, which gives the desired output at the second boundary, is accepted as the solution. In the proposed algorithm, the propagation from the initial boundary to the final one involves perturbations such as solar radiation pressure, Earth’s geopotential terms, solar and lunar gravitational forces. A root finding method based on bisection is used inside the shooting method. An admissible region originating from the semi-major axis is used in order to reduce the number of scenarios to be computed. In the original version of the OBVIOD, the initial orbit determination takes place by solving the Lambert’s problem. This version does not work well for the high area-to-mass (AMR) objects, especially over multiple revolutions. In this paper, OBVIOD’s performance is compared with the new shooting OBVIOD for different AMR values and varied number of revolutions between the boundaries. This comparison helps to identify the cases where it becomes important to make use of the proposed algorithm.
Preview