Multi-Hypothesis Light Curve Inversion Scheme for Convex Objects with Minimal Observations

Knowing the exterior shape forms an important piece of information for space debris in the near-Earth region. The shape is coupled with the object’s orbit via non-conservative forces and is integral to orbital predictions. It has been shown previously that the photometric measurements, also known as the light curve, contains such information. However, light curves are unresolved. This means a strong superposition of the aspects of shape, albedo, attitude, and viewing geometry is present. Furthermore, it has been shown that the problem cannot be posed to be unique. This is exacerbated in the presence of measurement noise.
For this work, a multi-hypothesis framework is proposed to solve the light curve inversion problem. The solution space is first sampled based on a primary observation dataset and the associated signal-to-noise ratio. Each candidate solution (a possible shape) then competes when follow-up observation is available. As a result, a list of most likely solutions can be sorted. The likeliness is determined by the correlation between the observation and the simulated measurements using candidate solutions. Without supplying additional measurements, the current effort is to combine likely shapes and form solutions with further credibility using geometry.
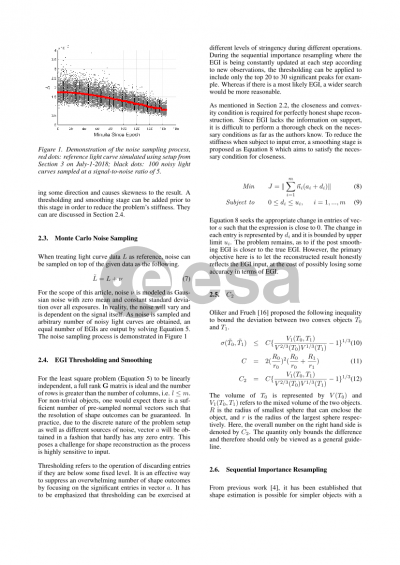
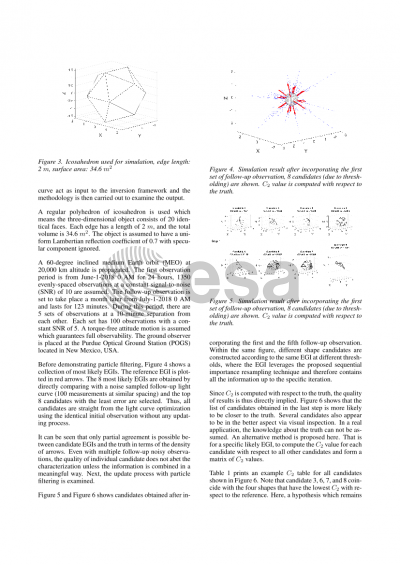
Results are demonstrated when subject to simulated input which is supplied by the in-house light curve simulation tool. The effect due to shape complexity, observation spacing, and noise, has been investigated. Four of the regular polyhedra with meter-level characteristic length are placed in Medium Earth Orbit. Three different data spacing are tested for the primary light curve, from 1350 to 2646 data points equally spaced within a 24-hour period. The signal-to-noise ratio also varies between 10 and 20. The condition for follow-up observation is restricted to 100 data points at an approximately 10-second interval with a signal-to-noise ratio of 5.
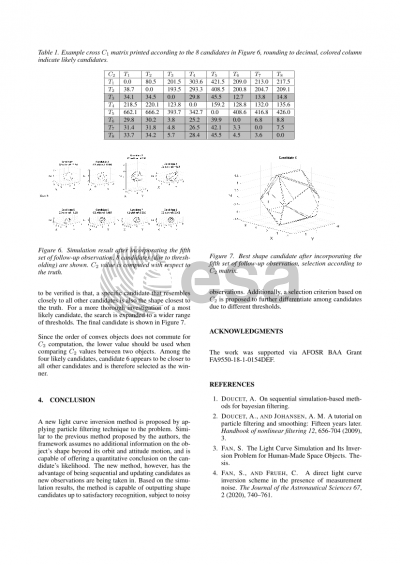
The closeness property of discrete Extended Gaussian Image has a significant impact on the shape retrieval process, thresholding remains to be the only effective way to maintain robustness when constructing the shape in the three-dimensional Euclidean space. As part of the work, two definitions (C_1 and C_2) are proposed to quantify the accuracy and closeness for such shape descriptor. In general, these two quantities can be used to predict the quality of the inversion. An additional smoothing stage is thus proposed based on the closeness property to reduce the stiffness during the shape construction process, where less skewness is observed, and more variety of answers is possible.
The simulation has shown that the proposed framework can offer a conclusive statement on possible exterior shapes of artificial objects with sharp edges, in terms of their likeliness. While only assuming knowledge on object’s attitude motion, using the framework can identify a list of likely shapes with partially correct features. The number of observations required for significant inversion results of test objects is on the level of thousands, which is ten to 100 times more compared to previous results.