A Novel Initial Orbit Determination Algorithm from Doppler and Angular Observations

Space Situational Awareness (SSA) refers to the ability to view, understand and predict the physical location of natural and manmade objects in orbit around the Earth. While the ability to view is made possible thanks to ground and space based sensors (e.g. Radars, Telescopes or Lasers) the ability to understand and predict the physical location of objects needs the determination of their orbital state vectors, which uniquely determine the trajectory of the object in space.
For the determination of the orbital state of an object, mainly two different situations have to be considered. Either the object is already known (i.e. it is regularly observed by sensors and an a priori state vector exists) or the object has been newly discovered by a so-called surveillance sensor. In the first case, the problem is already well known and the a-priori orbital state of the object can be updated thanks to the new gathered observations and a Least-Squares (LS) or an Extended Kalman Filter (EKF) filtering approach. In the second case, an a-priori orbital state have to be computed from the gathered observations, in order to predict the position of the newly detected object at short term and to improve the orbit thanks to the acquisition on new observations from surveillance and/or tracking sensors.
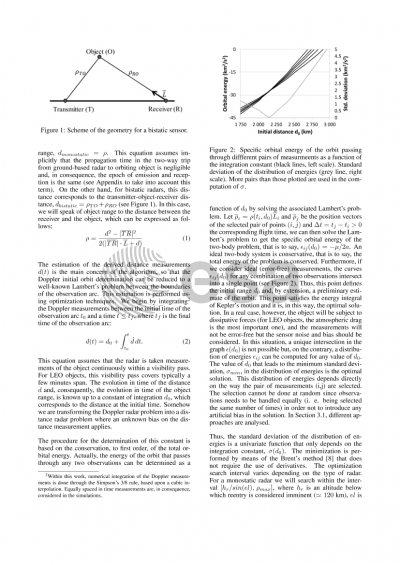
On this paper we interest ourselves to the second problem, and in particular we develop a novel numerical method for solving the initial orbit determination (IOD) problem in the frame of space debris surveillance systems. Differently from classical IOD methods, where three sets of angles ([1]-[3]) or two position vectors are used (i.e., the Lambert problem, [4]-[6]), the method presented in this paper makes use of N Doppler measurements along with their associated pairs of angular measurements coming from an Earth-based radar station. Numerical results are presented for objects in three different Low Earth Orbits showing the accuracy and performances obtained by this method.
References
[1] Vallado, D. A.: Fundamentals of Astrodynamics and Applications. Microcos Press, El Segundo, CA, Academic Publishers Dordrecht/Boston/Kluwer (2001)

[2] Escobal, P.R.: Methods of Orbit Determination. John Wiley and Sons, Inc., New York (1965)
[3] Gooding, R. H.: A new procedure for the solution of the classical problem of minimal orbit determination from three lines of sight. Celest. Mech. Dyn. Astr. 66(4), 387-423 (1997)

[4] Lancaster, E., Blanchard, R.: A unified form of Lambert's theorem. National Aeronautics and Space Administration. Technical Note D-5368. (1969)

[5] Gooding, R. H. : A procedure for the solution of lambert's orbital boundary-value problem. Celest. Mech. Dyn. Astr. 48(2), 145-165 (1990)

[6] Izzo, D.: Revisiting Lambert’s problem. Celest. Mech. Dyn. Astr. 121(1), 1-15 (2015)