On the Gaussianity validity time for orbital uncertainty propagation

Space Surveillance and Tracking (SST) aims to study and monitor resident space objects (RSO) and maintain the information about them within a catalogue. This includes the detection, tracking, cataloguing and identification of RSO, along with the analysis of the resulting catalogue for providing services such as collision risk assessment, fragmentation detection and identification as well as and reentry predictions. In many of the aspects of SST, the accuracy and realism of the object state uncertainty play an essential role. Measurements data association, performance of sensor tasking, correct estimation of probability of collision, to name a few examples, would fail or degrade severely if the orbital uncertainty is inaccurately represented.

The general assumption is that this state uncertainty can be represented by a multi-variate normal (MVN) distribution. However, in the frame of SST, the scarce of measurements due to a great number of space objects to track and the limited sensor resources, leads to the necessity of propagating the uncertainty during long periods of time. The intrinsic non-linear dynamics of space objects along with long propagation times yield to the breakdown of the Gaussianity assumption, and, therefore a single MVN is no longer a valid representation of the probability density function (PDF) of object state.

This works examines the validity time of the MVN representation in a twofold manner. Firstly, the use of statistical tests, namely the Royston-1992[1] procedure, to evaluate the departure from MVN assumption in the uncertainty distribution in Monte Carlo simulations. Secondly, the effect of non-linearity will be assessed by the comparison of orbital PDFs propagated with linear and non-linear methods. The measure of distance between both PDFs is evaluated with a scale-invariant form of the L2 distance. Linear propagation is performed with the usual state transition matrix (STM), whether the non-linear propagation makes use of higher order terms of the Taylor series linearization in the form of a state transition tensor (STT).

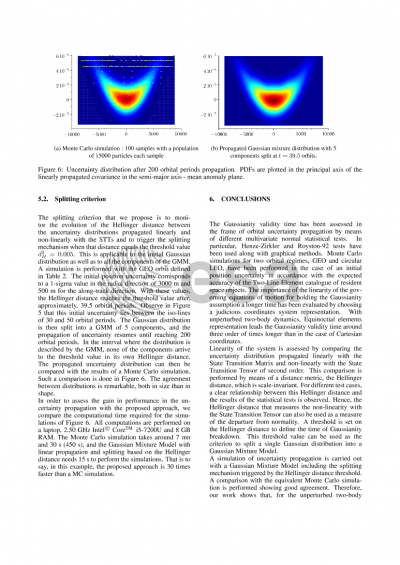
Both procedures are combined in order to link the departure from MVN representation to the importance of non-linear terms in the dynamics. Hence, it is possible to define a threshold in the normalized L2 distance that accounts for the Gaussianity validity time, and that would be the value to trigger the splitting mechanism for a Gaussian Mixture Model (GMM) representation. Finally, a practical example of GMM propagation using the splitting threshold defined in this work will be given.

[1] Royston, J.P., 1992. Approximating the Shapiro-Wilk W-Test for non-normality. Statistics and Computing, 2, 117-119.